This is the Second in a Set of Posts as a follow up to our ‘Introduction to Ganita’.

---

This Set of Posts on the Indic Perspective to Mathematics is the third installment of our continuing Series on Ganita.  Our first article in the Series celebrated Srinivasa Ramanujan. The Second provided an Introduction to Ganita. Emphases within quotes are ours.

Topic Outline

Part 1: (Introduction) 

In Part 1 of this Set of Posts on the Indic Perspective to Mathematics, we provided a background on the historical paradigms that drive the engines of Ganita and Western Mathematics respectively.

Part 2: (below) Ganita-Math Encounters. Ganita and Math came face-to-face when Indian Algorithms and Calculus traveled to Europe to help solve two critical problems: calculating with big numbers and managing the infinitely small. In a tense battle, Ganita’s balance of order and chaos prevails over the top-down Euclidean order backed by the church. We become aware of the massive contribution of the Vijayanagara empire to modern science.

Part 3: We adopt an Indic civilizational perspective of the Math-Ganita encounters. This gives rise to  interesting questions like ‘What was lost when Mathematics digested Ganita?’. We also look ahead, exploring the importance of Ganita and its Indian approach in a futuristic world.

Ganita-Mathematics Encounters

Experts have their expert fun
ex cathedra 
telling one 
just how nothing can be done. - Piet Hein.

In the Introduction to this Set of Posts, we studied the Greek origins of ‘Mathematics’. The abstract nature of Mathematics resulted in a drastically reduced practical output and Europe plunged into a 1000+ year dark era. During this period, Ganita contributions from Dharma thought systems helped keep math practically relevant in other parts of the world, right up to the 17-18th century CE. In particular, this injection of Ganita helped resolve two Math crises in Europe [1]. For the purpose of this post, we oversimplify and classify these problems as the ‘big’, and ‘small’ number’ crises. By helping resolve these crises, Ganita played a leading role in the birth and progress of modern science.

Big Number Crisis (Abacus vs Algorismus)

Here is example of a 10-digit Hindu number and its Roman numeral representation.

There are several such websites that allow us to perform this conversion and three aspects stand out. First is the reference to ‘Arab numbers‘ in many sites. Second, is a maximum limit on the input. Third, ‘0’ or negative numbers are not valid input. The idea of ‘Arab numbers’ is of course, deep-rooted in the western STEM community to this day (IEEE journal publication guidelines still refer erroneously to ‘Arabic numerals’) since a large body of Ganita knowledge made it to the west via Arab translations of Sanskrit works. As can be gauged from the conversion tool, the Roman system is cumbersome for doing actual calculations. Its representation is additive in nature and there is no place value for zero, and the idea that placing a ‘0’ after a number would increase its value was befuddling. The west relied on the abacus / counting board, which was adequate for simple arithmetic calculations (the Indians did most of their routine arithmetic mentally). The introduction of ‘algorismus’ from India via Arab sources  around the 11th-12th century CE provided the merchants of Florence with an incredibly advanced way of quickly and accurately performing all kinds of numerical calculations [1].

Although traders found it to be practically useful, resistance to the alien method was stiff and it was several centuries (16th century) before the Hindu system gained unanimous acceptance. Well, almost. The British treasury preferred to place their money in the ‘secure’ hands of the abacus and held out until the 17th century [1].  By that time, the second math crisis in Europe was well underway.

‘Smiling Boetius‘ works with Hindu numerals to prevail over his opponent, Pythagoras, who is sadly stuck with a counting board abacus. This depiction of the victory of ‘algorismus’ is on the cover of Gregor Reisch’s Margarita Philosophica (1508) [1].

Aside from the suspicion of an Arab source in a crusading world, a technical reason for the distrust appears to be Ganita’s approximation techniques combined with the fear of zeroes being added to make sums bigger. To a mind accustomed to the perfection of Euclidean math, not even the tiniest quantity could be discarded. Such unacceptable imperfections could open the door to fraud and chaos [1]. The Indian approach, since the Sulba Sutras, recognized the non-representability of certain quantities (e.g. √2) and employed pragmatic and epistemologically secure approximation methods without anxiety, in order to reduce uncertainty (round-off error) to within an acceptable level [1]. ‘Algorismus’ was absorbed into European practice in order to resolve real-life calculations, but not the underlying pramana and empirical rationale (e.g. upapatti).  Why?

Small Number crisis (Infinitesimals and the Indian Origin of Calculus)

The Indian Background Story

Brothers Harihara and Bukka, with the blessings of Rishi Vidyaranya, laid the foundation for one of the most important empires in Indian and world history in 1336 CE. In particular, the global scientific community owes the Vijayanagara empire a debt of gratitude.

While most regions of 14th century India reeled from the attack of fundamentalist invaders who had already destroyed India’s top universities and institutions, the Vijayanagara Empire became an oasis that protected and nurtured the Dharma. In particular, a school of Ganita was etablished in Kerala thanks to the prosperity and security enjoyed by the region during the Vijayanagara period, between the 14th and 16th century CE. An important member of this Ganita tradition was Madhava of Sangamagrama (~1350-1425 CE). This school produced a illustrious line of scholars who were the genuine adhyatmic and intellectual successors of Aryabhata, Bhaskara, and other great seekers. A major part of the foundation for modern science was laid by the Kerala school and the Ganita tradition they carried forward.

Recall that Aryabhata had already come up with finite difference equations for interpolation by 499 CE to generate fine-grained sine values. His practical approach essentially translates into Euler’s  18th century method for solving ordinary differential equations (ODEs). These results were subsequently improved upon by Brahmagupta (his second order interpolation result is known as ‘Stirling’s Interpolation Formula‘ today),  Bhaskara-2, and others [1]. Today, Indians are familiar with the phrase ‘Tatkal booking’ of train tickets. The ancient Ganita experts had developed algorithms  to calculate the Tatkalika gati of planets, their instantaneous velocity (an important quantity in Newtonian physics), as shown below.

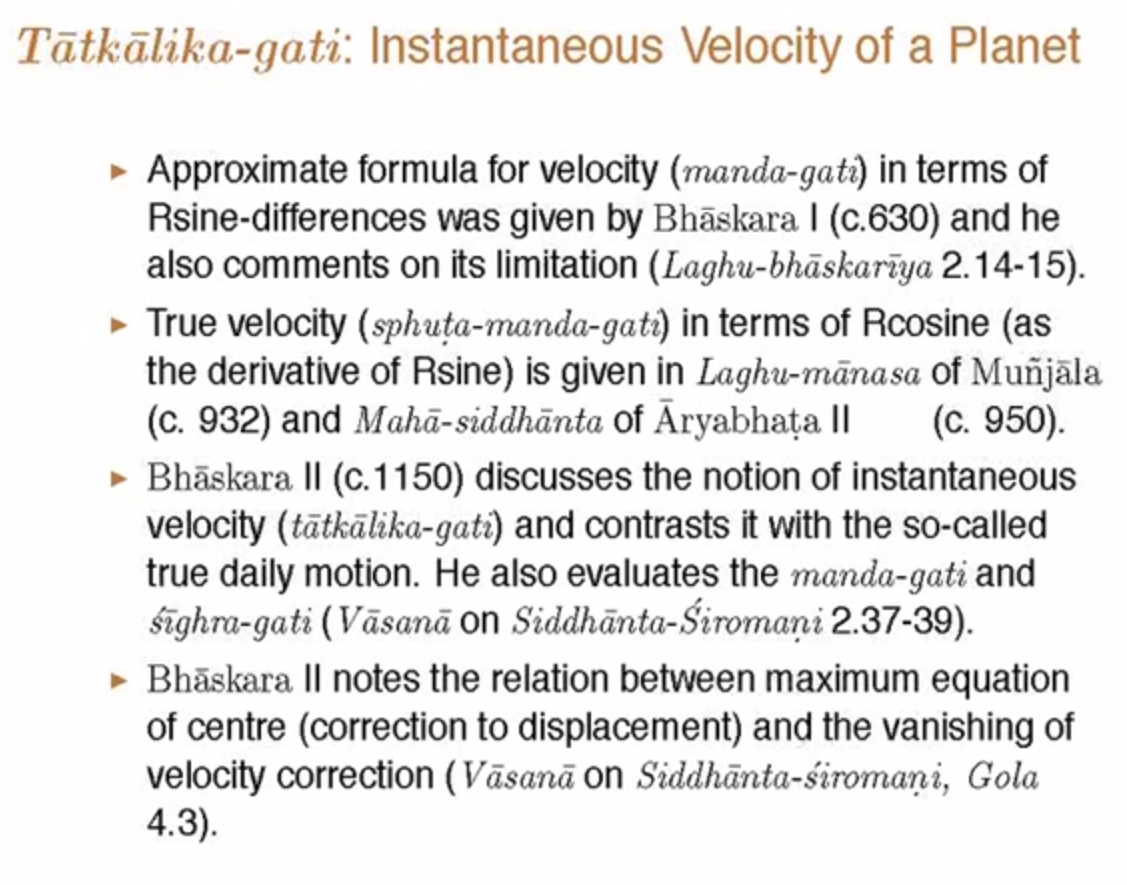
We can observe a continual progress in India toward calculus, right from Aryabhata [1]. For all practical purposes, the Ganita school in Kerala during the Vijayanagara period can rightfully claim to be the developers of Calculus (from a formal mathematics perspective, western historians credit them for ‘pre calculus’). C.K. Raju has demonstrated the all-around practical viability of this epistemologically secure calculus without the use of ‘limits’ [11].

Madhava gave the world some beautiful and important results in infinite series by 1375 CE, centuries before Newton/Leibniz/Gregory/Taylor/McLaurin & Co.

In the derivation of these calculus results we can observe a smart management of the non-representability of infinitesimals based on order counting, along with a judiciously chosen exceptional / end-correction term (right side of the picture above). This is a really cool and important innovation that serves twin purposes, as explained by C. K. Raju below [1].

There are many other novel ideas and instances of such Yukti within the Indian approach.  The interested reader can refer to [1] for a detailed description of the techniques employed.

It is worth comparing the meaningful Sanskrit non-translatable abhiyukti (expressing, or translating one’s Yukti in action) to its nearest English counterpart ‘algorithm’. The latter from the Latin ‘algorismus’, which in turn came from Al-khwarizmi who had translated Sanskrit texts of Ganita (see the picture at the top of this post). Jyesthadeva published the Ganita Yuktibhasa around 1530 CE in Malayalam, which provides the detailed mathematical rationale validating the Calculus results[1].

Why was Calculus Important to India?

Madhava’s infinite series with end-correction terms, allowed him to quickly calculate estimates for trigonometric values and π (pi) to very high levels of accuracy. For example, Madhava was able to calculate π to 11 decimal places, which represents both a quantitative, and methodological leap over prior brute-force type approaches (the next such dramatic leap was also due to Ganita, via Ramanujan) [14].  A natural follow-up question is: why were precise trigonometric values useful? Isn’t calculating π to many decimal places purely an academic exercise?  We summarize the reasons below, referring the interested reader to [1] for a detailed description.

Agriculture and Trade were key contributors to an Indian economy that played a dominant role on the world stage from 0 CE (and earlier) through 1750 CE.

Agriculture

1. Krishi was and is a dominant component of the Indian economy. It was (and still is) dependent on a successful rainy season, which means that accurately calculating the arrival time of monsoons is important. A couple of weeks ago, the Indian government announced a $60M supercomputer project to better predict monsoons.
2. Vedanga Jyotisha is primarily a science of time keeping that has numerous applications and has been recognized by researchers as a key source of knowledge in the ancient world [1]. It enabled the Indians to maintain an accurate calendar. Thus, from a Krishi perspective, the Ganita of Jyotisha acted as a decision support system for planning and scheduling key agricultural activities.
3. The Indian calendar date and time was calculated with respect to the prime meridian at Ujjain (long before Greenwich), which was then re-calibrated to obtain local times at locations all over Bharatvarsha that covered a vast area (ancient India was united by time too!). This local re-calibration:
   • ⇒ required the calculation of the local latitude and longitude (lat-long)
   • ⇒ which (in the Indian approach) used the size of Earth as input
   • ⇒ this required a value for π
   • ⇒ trigonometric values were also needed for lat-long calculations
   • Precise numerical values were required since tiny errors get magnified after multiplication by big numbers (in the order of the Earth’s radius). Thanks to the Ganita tradition, the Indians had access to good estimates that were continuously improved upon.

Overseas Trade

India has a culture of calculation and embodied knowing that goes back thousands of years. Many ordinary Indians even today take pride in their ability to think and calculate on their feet, or pull off some Jugaad without the aid of electronic devices. The pattern-seeking Indian nature is visible in their traditional approach to navigation, reflecting an ability to discover sufficient order even within an ocean of chaos. The metaphor of the Samudra Manthana truly comes alive here.

1. India, thanks to its manufacturing and technological prowess, had established lucrative trading relationships as a net exporter with several countries, from ancient Rome to the far east. Much was this was done through open sea routes, and not just sailing close to the coast [1].
2. Prior to the 11th century CE, accumulated navigational knowledge included seasonal wind patterns (‘wind lore’), nature of ocean currents (‘current lore’), etc., and the empirical wisdom of sea-craft. The ancient Tamizh seafarers made use of the Saptharishi mandalam (Ursa Major) in the southern hemisphere. This database of seafaring wisdom and best practices were preserved, improved upon, and transmitted from generation to generation via the oral traditions of the seafaring Jatis [27].
3. Thus, the Indian sailors had already established a tradition of navigation and deep sea voyage without written charts (they rejected the method of ‘dead reckoning‘ in order to stay alive). Their approach included an empirical understanding of ocean patterns, Ganita, and instrumentation like the rapalagai (kamal) for celestial observations. Tamizh navigators deciphered currents using a simple device known as mitappu palagai [27].
4. Such historical data further debunks the theory that oral traditions were ‘pre-rational’ and the sole preserve of Vedic scholars. Hinduphobic Indologists like Sheldon Pollock are dismissive of such priceless oral traditions [16]. The western universal idea of history begins with written text and it is tough for this mindset to imagine open-sea navigation without written charts.
5. Accurately determining the local lat-long using celestial observations (solar altitude at noon, pole star at night, etc.) was part of this approach.
6. More reliable navigation in the open seas is possible if the 3L: latitude, longitude, and loxodrome can be accurately obtained for any given location. These were indeed calculated in multiple ways by the Indians using trigonometric values [1].

Continual Progress in Calculating Accurate Trigonometric Values

1. Aryabhata’s astounding publication of his R-sine difference table along with an interpolation method stepped away from the geometrical approach that was employed until then [1]. The Aryabhatiya was a prized intellectual property of its time. It significantly improved the accuracy of trigonometric values (given the sine value of an angle, one can use elementary identities to calculate all other trig values).
2. Aryabhata’s work paved the way for Calculus. Over the next 1000 years, the Indians steadily improved upon prior estimates.
3. Calculus was a natural outcome of this process of deriving ever more accurate trigonometric values. The Kerala school’s calculus extended the finite series based trigonometric results to a highly accurate infinite series based approach.

We refer the reader to this essay [28] by D.P. Agarwal for his summary of the Kerala School, European Mathematics, and Navigation. It is highly likely that this Ganita knowledge traveled to Europe via European missionaries in Kerala and played a key part in revolutionizing physics and mechanics via Newton’s Principia Mathematica and other works.  This story serves as background for the question: why did the idea of ‘infinitesimals’ which was a non-issue in the Ganita world, spark a crisis in Europe?

The European Background Story

Ancient Greek math hit a roadblock after encountering paradoxes tied to infinitely small quantities. Mathematics could not deal with the irritating uncertainty around infinitesimals and the problem of non-representability: For example, an infinite number of threads of minuscule but nonzero length, joined end-to-end should yield an infinitely long thread. On the other hand, combining even an infinite number of threads of ‘zero’ length would only yield zero. Aristotle believed that continuum could be divided endlessly and could not be made up of ‘indivisibles’.

A famous paradox (which used to be popular among those preparing for engineering school entrance exams in India) is that of Achilles and the Tortoise. Around 500 BCE,  Zeno of Elea came up with several such paradoxes that exposed the gaps in a seemingly perfect mathematics and two-valued logic. Unable to satisfactorily resolve such contradictions and deal with non-representability of certain quantities (a fundamental requirement for numerical calculations), Greek progress halted. The dark ages robbed the west of native expertise and appears to have hurt them in key areas including, but not limited to [1, 27]:

• Astronomy, Navigation, Instrumentation
• Calendrical Systems, Ship Building
• Medicine and Botany

After more than a thousand years, between the 12th-16th century, we can observe the emergence of a new kind of Mathematics in Europe, which was fundamentally different in its epistemology from the Euclidean approach. This knowledge first arrived via Arab/Persian translations of Ganita works in Sanskrit, and later through Missionaries who had direct access to Ganita’s latest results in Sanskrit and local Indian languages. We kick off this discussion using the European calendar as a case study.

Trick question: What came after Thursday, October 4th, 1582 in Europe?

The answer is Friday, October 15th. The European (Julian) calendar was slow by about 11  minutes per year for about 1200 years across their dark age. Church and Biblical dogma reigned supreme from the time the Nicene creed was formalized in 325 CE. This dogma can be best understood as an instance of history-centrism [4], and a key to preserving the credibility of this ‘history’ of unique divine intervention is proper time keeping and dating of these events. This was a key motivation behind the European quest for a better calendar.

The Indians had maintained accurate calendars since ancient times thanks to Vedanga Jyotisha for use within multiple applications, and Buddhists even helped with calendars in China [1] (helping the Chinese is an old Indian habit…). The Roman Church realized in 1582 that their calendar was trailing the correct date by 11 whole days. This key project of calendar reform was taken up by Christopher Clavius (1538-1612 CE), a Jesuit priest. Thanks to his painstaking work, Pope Gregory was able to press the fast-forward button on the calendar (thereafter named after him), recommend a leap year correction, and the rest is history.

C.K. Raju has uncovered the Indian source of this calendar bug fix [24, 1]: “Jesuits, like Matteo Ricci, who trained in mathematics and astronomy, under Clavius’ new syllabus [Ricci also visited Coimbra and learnt navigation], were sent to India. In a 1581 letter, Ricci explicitly acknowledged that he was trying to understand local methods of timekeeping from “an intelligent Brahmin or an honest Moor”, in the vicinity of Cochin, which was, then, the key centre for mathematics and astronomy, since the Vijaynagar empire had sheltered it from the continuous onslaughts of raiders from the north. Language was hardly a problem, for the Jesuits had established a substantial presence in India, had a college in Cochin, and had even started  printing presses in local languages, like Malayalam and Tamil by the 1570’s.“. The Jesuits have continued to exercise their influence on the Indian education system to this day. They also played a key role in the second Math-Ganita tussle.

Jesuit (Euclidean) Order versus Indian (Ganita) Chaos

The Jesuits are members of the Society of Jesus, an organization founded by St. Ignatius of Loyola (1491-1556) and rooted in Roman Catholicism. Per [12] “In the broadest sense, imposing order on chaos was the Society’s core mission, both in its internal arrangements and in its engagement with the world.”

This painting of Ignatius of Loyola is richly symbolic. It depicts the victory of a perfect top-down hierarchical order over chaos. Loyola and his back-robed Jesuits are in the middle, watched over by angels at the top. Loyola is calmly performing an exorcism, expelling the chaotic evil spirits possessing the bodies of terrified people at the bottom of the picture. [12] provides an insightful description of this picture, noting the role of the black robed Jesuits of Loyola’s Society of Jesus: “They are Ignatius’s army, there to learn from their master, follow his directions, and ultimately take over his mission of turning chaos into order and bringing peace to the afflicted. For that was indeed the “miracle” of St. Ignatius and his followers. Like no one else, they managed to restore peace and order in a land torn apart by the challenge of the Reformation.“.

The Church gained immensely via this decisive mathematical triumph of calendar reform, and Clavius who played an instrumental role, realized the benefits obtainable by investing in mathematics. This was a time period characterized by fissures and dissent in Christianity, with several alternatives and reformations (e.g., led by Calvin) cropping up that challenged the exclusive authority of the catholic church. In this climate, Clavius felt that the top-down hierarchical perfection within Euclidean geometry would be a great fit for the Jesuit curriculum, and in sync with the primary goal of their founder St. Ignatius of Loyola.

As mentioned in [12] “It was clear to Clavius that Euclid’s method had succeeded in doing precisely what the Jesuits were struggling so hard to accomplish: imposing a true, eternal, and unchallengeable order upon a seemingly chaotic reality. Just as Ganita was recognized as the foremost of the sciences in India since ancient times, Euclidean Mathematics became a most important subject in Europe after the calendar reform. The Society of Jesus embraced Math and all was well for a while. The focus had shifted to other pressing topics. For example, navigational challenges had to be overcome in order to ‘discover‘ reliable sea routes to new lands.

The Indivisibles

Calculus created a rather sudden splash into Europe within 50 years of the calendar reform [1]. By that time, the calculus, which was rooted in Indian epistemology had already been developed and studied for two centuries.  Bonaventura Cavilieri (1598-1647), a Milanese Jesuat monk and a student of Galileo was an early adopter. While the Jesuits were more like a MNC, the Jesuats were a local group of Italian monks lower in the pecking order. However, Galileo’s endorsement boosted Cavalieri’s profile significantly. Cavalieri introduced the ‘method of indivisibles’, in which “planes and solids had an indeterminate number of indivisibles” and authored the book Geometria indivisibilibus (Geometry by Way of Indivisibles) in 1635 [12].

While the idea of indivisibles was embraced by the Galileans, the Jesuits were not as welcoming. Those who worked with infinitesimal quantities did so for its practical value in generating realistic new results and could not really establish any logical consistency needed to prove infallible theorems. Unlike Euclid’s Elements which used top-down deductive logic to prove specific theorems from axioms, the use of infinitesimals required the ground-up Ganita approach: to start from physical reality and work toward generalized results, which could lead to innovation and potentially unpredictable discoveries. Clearly, Yukti was not welcomed by the church whereas Galileo’s methods were more compatible with Ganita.

Galileo Galilei (1564 – 1642 CE)

Galileo had become a formidable opponent by that time. He had earlier discovered the moons around Jupiter, and as a prashasthi [16] to a rich grand duke who ruled Florence, named the moons after him and his family. In return, he was rewarded with benefits that included the post of ‘Chief Mathematician’ to the Duke in 1611, which also freed him up to pursue his work as an independent researcher. As [12] notes, “The Galileans also sought truth, but their approach was the reverse of that of the Jesuits: instead of imposing a unified order upon the world, they attempted to study the world as given, and to find the order within.” This started a conflict between the Galileans and the Jesuits.

For the church, the idea that matter could be broken down into infinitely small indivisible atoms was unacceptable. The archives of the Society of Jesus in Rome records for posterity the ruling of their leaders in 1632 on infinitesimals [12]:” “Judgment on the Composition of the Continuum by Indivisibles”. …The permanent continuum can be constituted of only physical indivisibles or atomic corpuscles having mathematical parts identified with them. Therefore the said corpuscles can be actually distinguished from each other.” The church basically ducked the question of non-representability and banned the idea and the mathematical study of ‘indivisibles’.

Among the critics of these indivisibles was Thomas Hobbes, the philosopher author of the Leviathan, who deeply influenced Western thought. Hobbes was also an excellent mathematician and a devotee of the Euclidean approach. He was bitterly opposed in this battle of the infinitesimals by John Wallis of England, one of the founders of the Royal Society, the new science academy [12]. Wallis had little time for eternal proofs, and was firmly rooted in what we can unmistakably recognize as the pragmatic Ganita approach for solving real-life problems. Hobbes had tried in vain for several years to prove that he could ‘square the circle‘, and each attempt in this futile exercise was eagerly demolished by Wallis and exploited to the hilt in their public feud [12]. Eventually, Wallis’ team ‘won’ the contest (possibly in terms of cultural and scientific acceptance) and Newton came up with his famous work Principia Mathematica that relies heavily on calculus. Interested readers can refer to [12, 1] for a detailed discussion.

The Ghosts of Departed Quantities

It is worth noting some logical inconsistencies in the positions of both sides in this battle. The church was fighting to save their dogmatic belief in an infallible and orderly Euclidean math against a group injecting a practically useful but poorly-understood imported concept into this math. Every researcher seemed to have his own pet model showing how the math of the infinitely small worked.  In an important and devastating piece of satirical writing, Anglican church bishop Berkeley ridiculed the questionable fluxions of Newton, and Leibniz’s ‘infinitesimal change’ as “the ghosts of departed quantities”. CK Raju concludes (as do others) that this calculus was not on firm epistemological ground.

The European approach appeared to be mechanical and did not, for example, employ the end-correction terms that had helped keep Indian derivation transparent and anchored in a valid pramana [1]. Mathematicians could not accept, understand, or were unaware of the Ganita rationale behind the amazing calculus results derived by the Kerala School. For example, it is known that “Newton later became discontented with the undeniable presence of infinitesimals in his calculus, and dissatisfied with the dubious procedure of “neglecting” them” [24].  Mathematics was enhanced so that calculus was eventually placed on a firm formal foundation in the 20th century [1].

Transmission of Calculus from India to Europe

The etymology of ‘calculus‘ (17th century CE, Latin) relates to ‘reckoning’ and ‘accounting’. This focus is entirely empirical and on calculation, far away from the Euclidean world of theorems and proofs. On the other hand, it is directly corresponds in meaning, intent, and usage to Ganita. So far, research has uncovered three kinds of evidence linking Indian Calculus transmission to Europe: documentary, circumstantial, and epistemological. The interested reader is referred to [24, 1] for details. A primary, initial motivation for appropriating Ganita’s calculus results appears to be the practical problem of navigation: to obtain accurate trigonometric values required to calculate the 3L mentioned earlier [1].

A note in [24] on the circumstantial evidence is worth stating: “Unlike India, where the series expansions developed over a thousand-year period 499-1501 CE, they appear suddenly in fully developed form in a Europe still adjusting to grasp arithmetic and decimal fractions“. The 1400+ year discontinuity in the study of infinitesimals  in Europe was followed by a sudden upsurge in results in the 16th-17th century [12], right after Ganita’s documented achievements in Kerala and the establishment of European missions along the west coast. In fact, this was also a period when results from Ayurveda and Siddha began traveling to Europe giving birth to modern Botany, and similarly revolutionizing western medicine, health-care, and sanitation.

Epistemological Evidence

The epistemological evidence is fascinating to read [1]. A barrier in the western mindset as far as dealing with uncertainty manifests itself clearly in both the first and second math crises. As noted in [24]: “The European difficulty with zero did not concern merely the numeral zero, but related also to the process of discarding or zeroing a “non-representable” during the course of a calculation—similar to the process of rounding. Though the Indian method of summing the infinite series constituted valid pramana, it was not understood in Europe; the earlier difficulty with non-representables zeroed during a calculation reappeared in a new form. This was now seen as a new difficulty—the problem of discarding infinitesimals… In both cases of algorismus and calculus, Europeans were unable to reject the new mathematical techniques because of the tremendous practical value for calculations (required for commerce, navigation etc.), and unable also to accept them because they did not fit in the metaphysical frame of what Europeans then regarded as valid“.

Another instructive story (see page 3 of this essay), highlighting the outcome and unintentional humor caused by a borrow-copy-paste of Ganita without fully understanding its epistemology, is about how ‘sine’ and ‘cosine’ entered Europe. These mistranslated terms destroy the insight behind the original Sanskrit terms jya and kojya [1], baffling generations of Indian students studying Trigonometry.

To this day, neither organized religion and its theology, nor secular mathematicians, have been able to fully embrace the epistemology and validation procedure of Ganita. Why is this? And examining this question from the other direction, why did the Indians not take Euclidean math seriously for two thousand years? What is the future of Ganita? We study these civilizational perspectives in the third and concluding Post of this Set.

Selected References

1. Cultural foundations of mathematics: the nature of mathematical proof and the transmission of the calculus from India to Europe in the 16th c. CE, C. K. Raju. Pearson Longman, 2007.
2. Plato on Mathematics. MacTutor History of Mathematics archive. 2007.
3. Plato’s Theory of Recollection. Uploaded by Lorenzo Colombani. Academia.edu. 2013.
4. Being Different: An Indian Challenge to Western Universalism. Rajiv Malhotra. Harper Collins. 2011.
5. Axiomatism and Computational Positivism: Two Mathematical Cultures in Pursuit of Exact Sciences. Roddam Narasimha. Reprinted from Economic and Political Weekly, 2003.
6. Use and Misues of Logic. Donald Simanek. 1997.
7. Computers, mathematics education, and the alternative epistemology of the calculus in the Yuktibhasa. C. K. Raju. 2001.
8.  American Veda: From Emerson and the Beatles to Yoga and Meditation How Indian Spirituality Changed the West. Phil Goldberg. Random House LLC. 2010.
9. Logic in Indian Thought. Subhash Kak.
10. Ramanujan’s Notebooks. Bruce Berndt. Mathematics Magazine (51). 1978.
11. C. K. Raju. Teaching mathematics with a different philosophy. Part 2: Calculus without Limits. 2013.
12. Infinitesimal: How a Dangerous Mathematical Theory Shaped the Modern World. Amir Alexander. Farrar, Straus and Giroux reprint / Scientific American. 2014.
13. Indra’s Net: Defending Hinduism’s Philosophical Unity. Rajiv Malhotra. Harper Collins. 2011
14. Mathematics in India – From Vedic Period to Modern Times: Video Lecture Series, by M. D. Srinivas. K. Ramasubramaniam, M. S. Sriram. 2013.
15. Mathematics Education in India: Status and Outlook. Editors: R. Ramanujam, K. Subramaniam. Homi Bhabha Centre for Science Education, TIFR. 2012.
16. The Battle For Sanskrit. Rajiv Malhotra. Harper Collins. 2016.

(A full list of references will be published along with Part-3).

Acknowledgment: Big thanks to the ICP blogger and the editor for their constructive feedback, patience, and comments that helped shape and improve this post.